Roughness

Roughness can be predicted from the distribution of permanent deformation using the approach described below:

The standard deviation of the rut depth (permanent deformation at the surface), σ, may be found from:

where         rdi is the rut depth at point i,

N is the number of points, and

μ is the average rut depth.

The autocorrelation coefficient, ρ, is the correlation coefficient of consecutive points at constant distances. It may be calculated from:

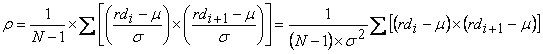
Slope Variance (SV) was used at the AASHO Road Test to describe roughness. The slope is measured, in mm/m, over a distance of 300 mm. The Slope Variance may be calculated from:

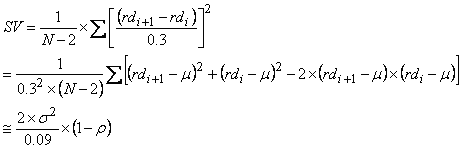
This means that the roughness, in terms of slope variance, is determined by the standard deviation of the rut depth and the autocorrelation coefficient for a distance of 300 mm.

A longitudinal surface profile that has a standard deviation of s and an autocorrelation coefficient of r can be generated through a first order autoregressive process:

where xt is the elevation at point t and at is a normally distributed random variable with mean value 0 and a standard deviation of sx.

With CalME it is possible to use a second order autoregressive process:

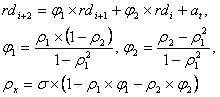
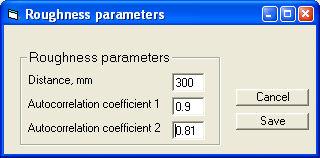
The parameters are entered in the form below, through the menu points "Special input" and "Change Roughness Parameters":

The second autocorrelation coefficient r2 should be less than or equal to the square of the first autocorrelation coefficient  (if equal the process reverts to a first order autoregressive process).

The standard deviation, s, is calculated from the variation in predicted rut depth and a longitudinal surface profile is generated using the distance and autocorrelation coefficients. The "Golden Car" (Sayers & Karamihas, 1998) is used to calculate the IRI values from this profile.

When variability is considered the IRI value will also be reported in the output, as shown in Example_2.xls.