Asphaltic Stiffness Master Curve

For asphaltic bound materials the modulus is determined from a model of the format used in MEPDG:

where E is the modulus in MPa,

 tr is reduced time in sec,

 α, β, γ, and δ are constants, and

 logarithms are to base 10.

Reduced time is found from:

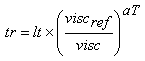
where lt is the loading time (in sec)

  viscref is the binder viscosity at the reference temperature T_ref,

 visc is the binder viscosity at the present temperature, and

 aT is a constant.

The viscosity is found from:

where TK is the temperature (in °K), and

 A and VTS are constants.

To get the loglog linear relationship of the equation the viscosity must be given in cPoise. The SI unit for viscosity is Pa•sec (= 10 Poise = 1000 cPoise = 10 Stoke (St)).

For asphaltic materials the temperature is calculated at a depth one third into the material and the loading time is calculated as 200 mm plus the depth one third into the material, divided by the wheel speed in [200 + z]/speed.

Examples of the viscosity parameters are shown below, given for temperature in ºR (A ºK = A ºR + VTS×log(9/5)). A given mix modulus versus temperature relationship can, however, be fitted quite well with a number of different viscosity versus temperature relationships.

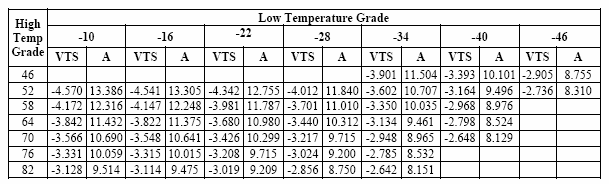
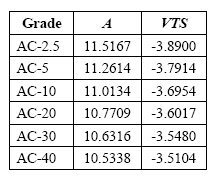
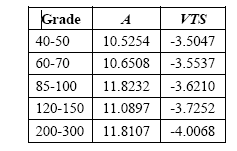
Viscosity susceptibility parameters recommended by MEPDG for PG, viscosity and penetration grades.

The figure below shows the results of frequency sweep tests done at three different temperatures (15, 19 and 28 oC)

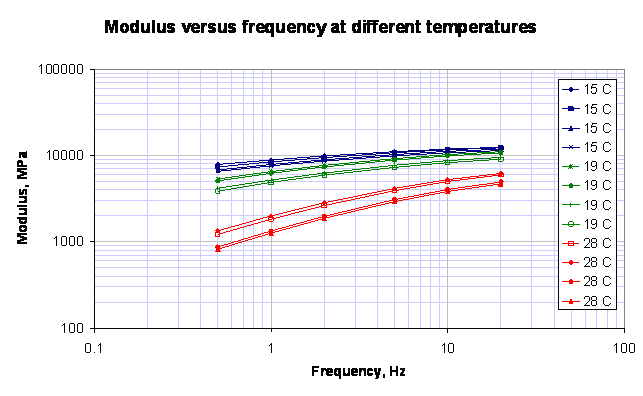
It was assumed that the binder viscosity could be described by A = 9.6307 and VTS = -3.5047 (temperatures in degree Kelvin). The master curve is not sensitive to the selection of binder parameters and almost any of the combinations given in the tables above could have been used.

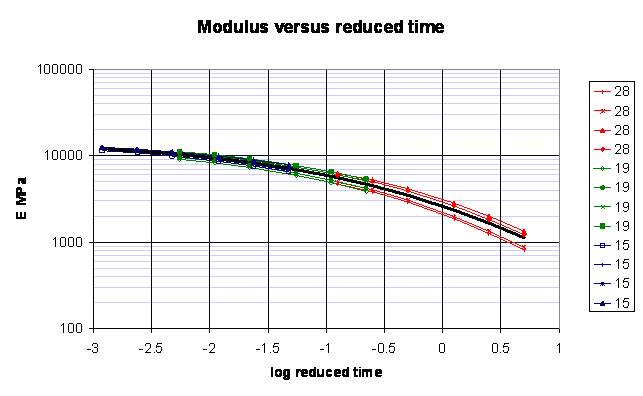
The measured moduli were fitted to the master curve equation using Solver in an Excel spreadsheet and minimizing the Root Mean Square (RMS) difference between the measured values and the values calculated from the master curve equation. In the following figure the measured moduli are plotted against reduced time. The heavy black line is the master curve equation.

The master curve equation for this case was:

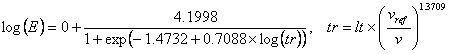
In this case the minimum modulus was assumed to be 1 MPa in order to fit the measured moduli. For use with CalME a minimum modulus of 200 MPa, corresponding to the modulus of the aggregate alone, is recommended even though it may not fit the softer part of the laboratory data as well.